Fermat's Little Theorem
August 1st, 2018
Reflections on 'Prime number mysteries' an article written by Johan P. Hansen (1915) Aarhus University:
Download PDF
The Absolute q-values are Decreasing
August 20th, 2017
In this article I show that the absolute q-values are decreasing.
Download PDF
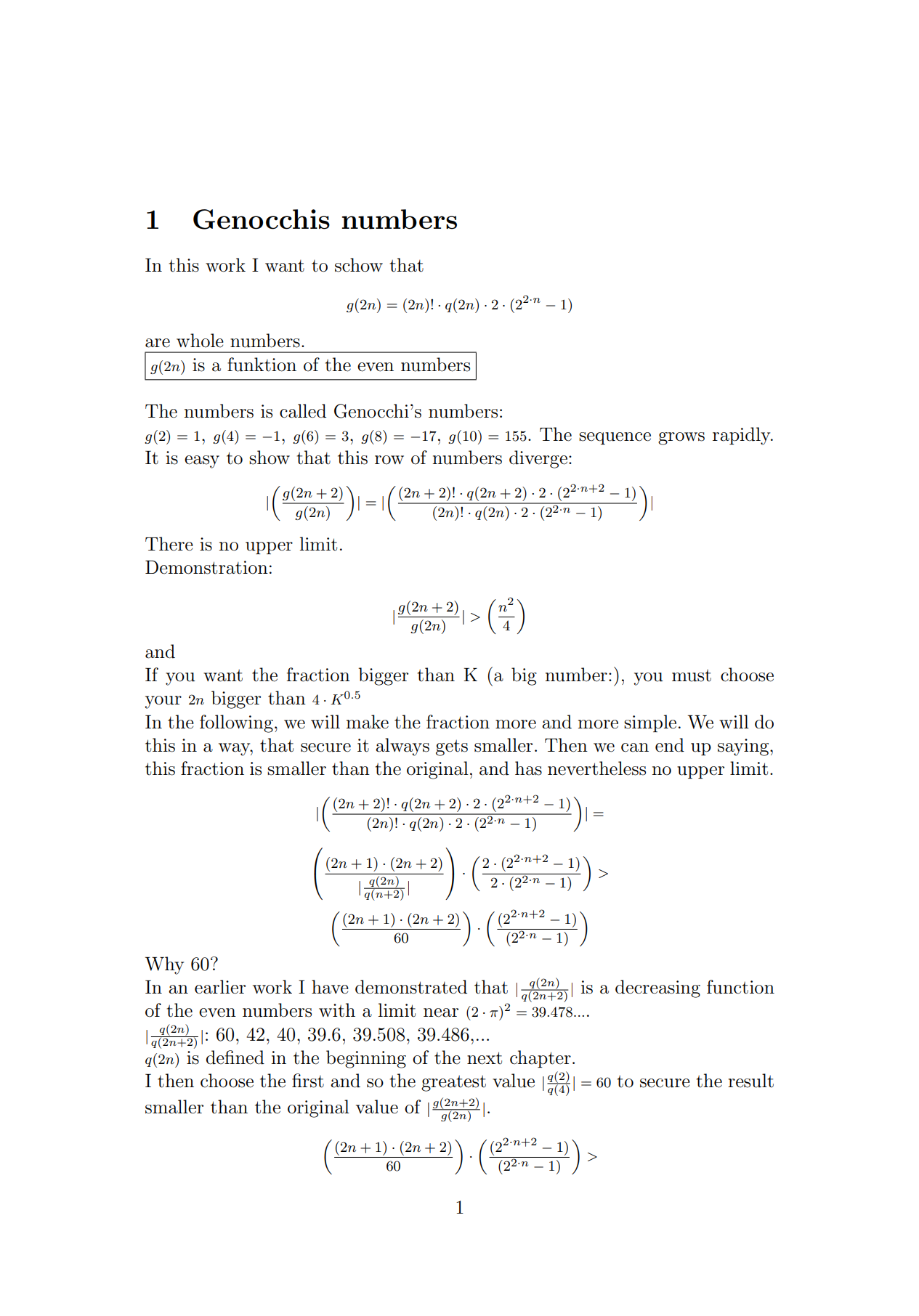
Genocchi's Numbers
June 29th, 2017
In this work I want to show that:
$ g(2n) = (2n)! · q(2n) · 2 · (2^{2n} − 1) $
are whole numbers.
Download PDF
Shorter Proof that q-value Fractions are Decreasing
January 29th, 2009
The case of a shorter and more straight forward proof that the fractions:
$ \frac{q_(2t) }{ q_(2t + 2)} $
are decreasing.
Download PDF
The Monster Fractions continued (part 2)
December 9th, 2008
Look at this difference:
$\delta_{s}=\left(\left|\frac{q_{(2\cdot s)}}{q_{(2\cdot
s+2)}}\right|-\left|\frac{q_{(2\cdot t)}}{q_{(2\cdot
t+2)}}\right|\right)\cdot\frac{|q_{(2\cdot t+2)}|}{|q_{(2\cdot t)}|}\cdot
|q_{(2\cdot s+2)}|\cdot |q_{(2\cdot t-2\cdot s)}|$
$-\left(\left|\frac{q_{(2\cdot s)}}{q_{(2\cdot
s+2)}}\right|-\left|\frac{q_{(2\cdot t)}}{q_{(2\cdot
t+2)}}\right|\right)\cdot\frac{|q_{(2\cdot t+2-2\cdot s)}|}{|q_{(2\cdot t-2\cdot s)}|}\cdot
|q_{(2\cdot s+2)}|\cdot |q_{(2\cdot t-2\cdot s)}| $
which can be written as this product:
$\delta_{s}=\left(\left|\frac{q_{(2\cdot s)}}{q_{(2\cdot
s+2)}}\right|-\left|\frac{q_{(2\cdot t)}}{q_{(2\cdot
t+2)}}\right|\right) \cdot \left(\left|\frac{q_{(2\cdot t+2)}}{q_{(2\cdot
t)}}\right|-\left|\frac{q_{(2\cdot t+2-2\cdot s)}}
{q_{(2\cdot t-2\cdot s)}}\right|\right)\cdot|q_{(2\cdot s+2)}|\cdot |q_{(2\cdot t-2\cdot s)}| $
It is about my q-values, which is a function of the natural numbers and zero. Here we are
focused on the even numbers.
The function of the even numbers are producing a series of small rational
numbers the numeric values of which are decreasing as t approaches infinity
exponentially much
like the values of a function such as $(\frac{1}{40})^n$.
I constructed this $\delta_s$-product (or rather it materialized out of the
blue) in the battle of proving that the fraction $\left|\frac{q_{(2\cdot
t)}}{q_{(2\cdot
t+2)}}\right|$ was falling too
The proof turned out to be a rather lengthy affair. It took me 18 pages to convince
myself that I had got it right. It was a reductio ad absurdum-proof:
if the
function is not decreasing 2 might as well be 4 - you know.
I have never used that kind of proof before even though I admire Euclid's famous
proof that there can't be any greatest prime number.
Since I finished the proof I have had this feeling that I must be able to do a little
shorter and more straightforward.
For some time actually for a couple of years, I have tried to prove that what I
call the the monsterfraction is decreasing too:
The monsterfraction:
$\frac{\left|\frac{q_{(2\cdot
t-2)}}{q_{(2\cdot
t)}}\right|}{\left|\frac{q_{(2\cdot
t)}}{q_{(2\cdot
t+2)}}\right|}$
Long time ago I proved that if the series of the smaller fractions $\left|\frac{q_{(2\cdot
t-2)}}{q_{(2\cdot
t)}}\right|$ were decreasing then the monsters had the limit 1, but even though they
are all bigger than one
since
$\left|\frac{q_{(2\cdot
t-2)}}{q_{(2\cdot
t)}}\right|>\left|\frac{q_{(2\cdot
t)}}{q_{(2\cdot
t+2)}}\right|$
there are different ways of reaching 1 than clean
falling as I have explained earlier.
It is a little like the cat trying to catch its tail.
But if the monsters are strictly
decreasing towards 1, our smaller fraction must get more and more close to
each other. Packed together in the correct order.
In other words they will have a common limit.
Now let us return to the $\delta_s$-product and the case of a shorter and more
straightforward proof that $\left|\frac{q_{(2\cdot
t)}}{q_{(2\cdot
t+2)}}\right|$ is a decreasing series:
$\delta_{s}=\left(\left|\frac{q_{(2\cdot s)}}{q_{(2\cdot
s+2)}}\right|-\left|\frac{q_{(2\cdot t)}}{q_{(2\cdot
t+2)}}\right|\right) \cdot \left(\left|\frac{q_{(2\cdot t+2)}}{q_{(2\cdot
t)}}\right|-\left|\frac{q_{(2\cdot t+2-2\cdot s)}}
{q_{(2\cdot t-2\cdot s)}}\right|\right)\cdot|q_{(2\cdot s+2)}|\cdot |q_{(2\cdot t-2\cdot s)}| $
It is rather laborious calculations, but we could let Maple affirm for all s greater than zero
up to a chosen t (let us say $t=10$), that the
first difference in the product is positive, or what is actually the same
thing, that $\left|\frac{q_{(2\cdot
s)}}{q_{(2\cdot
s+2)}}\right|$ is falling as long as $0 < s < t$
Now the second factor in the product must be positive too, since $a>b$ means that
$\frac{1}{b}>\frac{1}{a}$
Could we get away with letting s be equal to zero and later equal to t? Well it would not
make much difference since in both cases the product will be zero.
The last 2 factors are secured to be positive by the numeric signs, so the
product will be positive or zero at least until s reaches t.
What about the difference between the next 2 fractions:
$\left|\frac{q_{(2\cdot t)}}{q_{(2\cdot
t+2)}}\right|-\left|\frac{q_{(2\cdot t+2)}}{q_{(2\cdot
t+4)}}\right|$
Well we could add all these $\delta_s$-products in a sum and call it big delta:
$\Delta_{q_{(2\cdot t+2)}}=\sum_{s=0}^{t}\delta_{s}$
And I can show that
$\left(
\left|\frac{q_{(2\cdot t)}}{q_{(2\cdot t+2)}}\right|-\left| \frac{q_{(2\cdot
t+2)}}{q_{(2\cdot t+4)}}\right|\right)\cdot(2\cdot t+5)\cdot|q_{2\cdot t+4}|=\Delta_{q_{(2\cdot t+2)}}$
or
$\left(
\left|\frac{q_{(2\cdot t)}}{q_{(2\cdot t+2)}}\right|-\left| \frac{q_{(2\cdot
t+2)}}{q_{(2\cdot t+4)}}\right|\right)=\frac{\Delta_{q_{(2\cdot t+2)}}}{(2\cdot t+5)\cdot|q_{2\cdot
t+4}|}$
$\left|\frac{q_{(2\cdot t)}}{q_{(2\cdot
t+2)}}\right|$ must be bigger than $\left|\frac{q_{(2\cdot t+2)}}{q_{(2\cdot
t+4)}}\right|$ since the difference is the sum of positive numbers divided by a
positive product.
Which was to be proved.
This is certainly shorter and more simple than the original proof.
It is a classical proof of induction
Yes I still need to show the last step. I will simply steal it from my old lengthy proof. I will come back to that, but at first
we will return to the $\delta_s$-products or rather to the difference before it:
$\delta_{s}=\left(\left|\frac{q_{(2\cdot s)}}{q_{(2\cdot
s+2)}}\right|-\left|\frac{q_{(2\cdot t)}}{q_{(2\cdot
t+2)}}\right|\right)\cdot\frac{|q_{(2\cdot t+2)}|}{|q_{(2\cdot t)}|}\cdot
|q_{(2\cdot s+2)}|\cdot |q_{(2\cdot t-2\cdot s)}|$
$-\left(\left|\frac{q_{(2\cdot s)}}{q_{(2\cdot
s+2)}}\right|-\left|\frac{q_{(2\cdot t)}}{q_{(2\cdot
t+2)}}\right|\right)\cdot\frac{|q_{(2\cdot t+2-2\cdot s)}|}{|q_{(2\cdot t-2\cdot s)}|}\cdot
|q_{(2\cdot s+2)}|\cdot |q_{(2\cdot t-2\cdot s)}| $
because there may be more than one story to be told.
The Monster Fractions continued (part 1)
January 24th, 2008
In the summer 2006 I spent a week's holiday in the cottage of Rosenholm in
Smaaland in Sweden.
During that time I made this proposition with a little help from Maple. I found it very
interesting,
maybe I found it more interesting, than it deserves. But it served me well for some time.
I called it The
Rosenholm Model:
$\left(\frac{q(n-2)}{q(n)}\cdot\frac{q(n)}{q(n+2)}\right)^{\frac{1}{2^{(n+2)}}}<\left(\frac{\frac{q(n-2)}{q(n)}}{\frac{q(n)}{q(n+2)}}\right)<\left(\frac{q(n-2)}{q(n)}\cdot\frac{q(n)}{q(n+2)}\right)^{\frac{1}{2^{(n+1)}}}$
If this hypothesis is correct, it must too be true that:
$\left(\left(\frac{q(n-2)}{q(n)}\cdot\frac{q(n)}{q(n+2)}\right)^{\frac{1}{2^{(n+2)}}}\right)^4<\left(\frac{\frac{q(n-2)}{q(n)}}{\frac{q(n)}{q(n+2)}}\right)^4<\left(\left(\frac{q(n-2)}{q(n)}\cdot\frac{q(n)}{q(n+2)}\right)^{\frac{1}{2^{(n+1)}}}\right)^4$
and this:
$\left(\frac{q(n-2}{q(n)}\cdot\frac{q(n)}{q(n+2)}\right)^{\frac{1}{2^{(n)}}}<\left(\frac{\frac{q(n-2)}{q(n)}}{\frac{q(n)}{q(n+2)}}\right)^4<\left(\frac{q(n-2)}{q(n)}\cdot\frac{q(n)}{q(n+2)}\right)^{\frac{1}{2^{(n-1)}}}$.
The following product must be bigger than 1:
$\left(\frac{\frac{q(n-4)}{q(n-2)}}{\frac{q(n-2)}{q(n)}}\cdot\frac{\frac{q(n-2)}{q(n)}}{\frac{q(n)}{q(n+2)}}\right) $
And therefore this must be right:
$\left(\frac{q(n-2)}{q(n)}\cdot\frac{q(n)}{q(n+2)}\right)<\left(\frac{q(n-2)}{q(n)}\cdot\frac{q(n)}{q(n+2)}\right)\cdot\left(\frac{\frac{q(n-4))}{q(n-2)}}{\frac{q(n-2)}{q(n)}}\cdot\frac{\frac{q(n-2)}{q(n)}}{\frac{q(n)}{q(n+2)}}\right)=\left(\frac{q(n-4)}{q(n-2)}\cdot\frac{q(n-2)}{q(n)}\right)$
We may now tentatively write:
$\left(\frac{q(n-2)}{q(n)}\cdot\frac{q(n)}{q(n+2)}\right)^{\frac{1}{2^{(n)}}}<\left(\frac{q(n-4)}{q(n-2)}\cdot\frac{q(n-2)}{q(n)}\right)^{\frac{1}{2^{(n)}}}<\left(\frac{\frac{q(n-2)}{q(n)}}{\frac{q(n)}{q(n+2)}}\right)^4<\left(\frac{\frac{q(n-4)}{q(n-2)}}{\frac{q(n-2)}{q(n)}}\right)<\left(\frac{q(n-2)}{q(n)}\cdot\frac{q(n)}{q(n+2)}\right)^{\frac{1}{2^{(n-1)}}}$
$<\left(\frac{q(n-4)}{q(n-2)}\cdot\frac{q(n-2)}{q(n)}\right)^{\frac{1}{2^{(n-1)}}}$
We could raise every expression to the power of $2^{n}$:
$\left(\frac{q(n-2)}{q(n)}\cdot\frac{q(n)}{q(n+2)}\right)<\left(\frac{q(n-4)}{q(n-2)}\cdot\frac{q(n-2)}{q(n)}\right)<\left(\frac{\frac{q(n-2)}{q(n)}}{\frac{q(n)}{q(n+2)}}\right)^{2^{n+2}}<\left(\frac{\frac{q(n-4)}{q(n-2)}}{\frac{q(n-2)}{q(n)}}\right)^{2^{n}}<\left(\frac{q(n-2)}{q(n)}\cdot\frac{q(n)}{q(n+2)}\right)^{2}$
$<\left(\frac{q(n-4)}{q(n-2)}\cdot\frac{q(n-2)}{q(n)}\right)^{2}$
And extrapolate:
$\left(\frac{q(n-2)}{q(n)}\cdot\frac{q(n)}{q(n+2)}\right)<\left(\frac{q(n-4)}{q(n-2)}\cdot\frac{q(n-2)}{q(n)}\right)<\ldots<\left(\frac{q(4)}{q(6)}\cdot\frac{q(6)}{q(8)}\right)<$
$\left(\frac{\frac{q(n-2)}{q(n)}}{\frac{q(n)}{q(n+2)}}\right)^{2^{n+2}}<\left(\frac{\frac{q(n-4)}{q(n-2)}}{\frac{q(n-2)}{q(n)}}\right)^{2^{n}}<\ldots<\left(\frac{\frac{q(4)}{q(6)}}{\frac{q(6)}{q(8)}}\right)^{2^{8}}$
$<\left(\frac{q(n-2)}{q(n)}\cdot\frac{q(n)}{q(n+2)}\right)^{2}
<\left(\frac{q(n-4)}{q(n-2)}\cdot\frac{q(n-2)}{q(n)}\right)^{2}<\ldots<\left(\frac{q(4)}{q(6)}\cdot\frac{q(6)}{q(8)}\right)^2$
The Rosenholm Model lead us to the same conclusion:
$\left(\frac{\frac{q(n-2)}{q(n)}}{\frac{q(n)}{q(n+2)}}\right)^{2^{n+2}}<\left(\frac{\frac{q(n-4)}{q(n-2)}}{\frac{q(n-2)}{q(n)}}\right)^{2^{n}}<\ldots<\left(\frac{\frac{q(4)}{q(6)}}{\frac{q(6)}{q(8)}}\right)^{2^{8}}<\left(\frac{\frac{q(2)}{q(4)}}{\frac{q(4)}{q(6)}}\right)^{2^{6}}$
The row of monsters raised from the power of $2^{6}$ to $2^{n+2}$:
$\left(\frac{\frac{q(n-2)}{q(n)}}{\frac{q(n)}{q(n+2)}}\right)^{2^{n+2}}$
seems to be decreasing and as $n$ gets bigger, it gets suspiciuosly close to
$e^{9}=8103.08392\ldots$
for $n=60$ it is $8103.08393\ldots$
What about the expressions?
$\left(\frac{q(n-2)}{q(n)}\cdot\frac{q(n)}{q(n+2)}\right)$ and
$\left(\frac{q(n-2)}{q(n)}\cdot\frac{q(n)}{q(n+2)}\right)^{2}$
They are falling too and very soon getting suspiciuosly close to
$(2\cdot \pi)^{4}=1558.545456544038\ldots$
and
$(2\cdot \pi)^{8}=2429063.9401140669\ldots$.
This matches the numerical values of the expressions for $n=60$.
$e^{9}$ and all the corresponding monsters except the first are between them.
This is no big deal, not really interresting though I thought so at the time,
I can place the blame on Maple :)
I have just stumbled into
two expressions: $\left(\frac{q(n-2)}{q(n)}\cdot\frac{q(n)}{q(n+2)}\right)$ and
$\left(\frac{q(n-2)}{q(n)}\cdot\frac{q(n)}{q(n+2)}\right)^{2}$
, which soon get near fixed numbers:
$(2\cdot \pi)^{4}$ and $(2\cdot \pi)^{8}$
and placed conveniently on each
side of the row.
I could have picked the numbers: $2000$ and $10000$, and they
would have fitted just as well or better.
I first realized this when I raised all expressions to the power of
$2^{n+2}$.
What about $\left(\frac{\frac{q(n-2)}{q(n)}}{\frac{q(n)}{q(n+2)}}\right)$ getting
$1$ in the limit?
Well, it looks like that we now have this row:
$e^9<\ldots\left(\frac{\frac{q(n-2)}{q(n)}}{\frac{q(n)}{q(n+2)}}\right)^{2^{n+2}}<\left(\frac{\frac{q(n-4)}{q(n-2)}}{\frac{q(n-2)}{q(n)}}\right)^{2^{n}}<\ldots<\left(\frac{\frac{q(4)}{q(6)}}{\frac{q(6)}{q(8)}}\right)^{2^{8}}<\left(\frac{\frac{q(2)}{q(4)}}{\frac{q(4)}{q(6)}}\right)^{2^{6}}$
If we raise all expressions to the power of$\frac{1}{2^{(n+2)}}$ we will get:
$(e^9)^{\frac{1}{2^{(n+2)}}}<\ldots\left(\frac{\frac{q(n-2)}{q(n)}}{\frac{q(n)}{q(n+2)}}\right)<\left(\frac{\frac{q(n-4)}{q(n-2)}}{\frac{q(n-2)}{q(n)}}\right)^{\frac{1}{2^{2}}}<\ldots<\left(\frac{\frac{q(4)}{q(6)}}{\frac{q(6)}{q(8)}}\right)^{\frac{1}{2^{n-6}}}<\left(\frac{\frac{q(2)}{q(4)}}{\frac{q(4)}{q(6)}}\right)^{\frac{1}{2^{n-4}}}$
and $\left(\frac{\frac{q(n-2)}{q(n)}}{\frac{q(n)}{q(n+2)}}\right)$ will not only approach $1$ pretty
fast, but approach each other even faster:
$(e^9)^{\frac{1}{2^{(n+2)}}}=1.0000000000000000019515639104\ldots$
matching for $n=60$
$\left(\frac{\frac{q(n-2)}{q(n)}}{\frac{q(n)}{q(n+2)}}\right)=1.0000000000000000019515639106\ldots$
$(e^9)^{\frac{1}{2^{(n+2)}}}=e^{\frac{9}{2^{(n+2)}}}$
$\frac{9}{2^{(n+2)}}\rightarrow 0, n\rightarrow \infty $
and $e^0=1$
By the way
for $n=60$
$\left(\frac{\frac{q(2)}{q(4)}}{\frac{q(4)}{q(6)}}\right)^{\frac{1}{2^{n-4}}}=1.0000000000000000049$
$e^{\frac{9}{2^{(n+2)}}}=1.0000000000000000019$
The Monster Fractions
November 20, 2007
I have done some cleaning up in my mathematical projekt about the q-values:
It concerns this fraction:
$\frac{\left(\frac{q(n-2)}{q(n)}\right)}{\left(\frac{q(n)}{q(n+2)}\right)}$
Why would anyone be interested in this monster?
Well, I am, because if the limit is 1,
the reason must be that the two fractions in the monster:
$\left(\frac{q(n-2)}{q(n)}\right)$ and $\left(\frac{q(n)}{q(n+2)}\right)$
are
getting more and more equal when n gets bigger.
In other words the fraction:
$\left(\frac{q(n-2)}{q(n)}\right)$
has a limit value for
$n\rightarrow \infty$
.
Last Christmas (2006) I proved this to be the case under the condition that
$\left(\frac{q(n-2)}{q(n)}\right)$ was a falling function (getting smaller for
bigger n). A condition which I finally proved in the spring this year.
Now the stage should be set to prove what the limit of this fraction is.
The first
fraction in the row $\left(\frac{q(2)}{q(4)}\right)$ being 60 and the "last" are bigger than 39 (proven, and I can do better than that).
Before I start on that project I would like to prove that the monster too is
falling when n gets bigger.
You may think it has to be falling always being bigger than 1 because
$\left(\frac{q(n-2)}{q(n)}\right)>\left(\frac{q(n)}{q(n+2)}\right)$ and never the less ending up
being 1 in the limit.
But it don't has to get there falling every time n grows to
(n+2).
The graph could look like a staircase. Values of the monsters being the same
for a couple of n's then fall.
Or it could look like the graph for sales numbers in directors office in a firm not going too
good. Every now and then the director says: "We are doing better now", but next month
the graph drops again and lower than ever before.
Maple suggests, that the monster falls in such a way, that the next value is
lesser than the fourth root of it:
$\frac{\left(\frac{q(n-2)}{q(n)}\right)}{\left(\frac{q(n)}{q(n+2)}\right)}<\left(\frac{\left(\frac{q(n-4)}{q(n-2)}\right)}{\left(\frac{q(n-2)}{q(n)}\right)}\right)^{(\frac{1}{4})}$
And that is falling since the monsters are all bigger than 1.
Had they been smaller than 1, they would get bigger, when you take the fourth root
of them.
The inequality above could be extrapolated backwards to the start of the
monsters:
$ 1<\frac{\left(\frac{q(n-2)}{q(n)}\right)}{\left(\frac{q(n)}{q(n+2)}\right)}<\left(\frac{\left(\frac{q(n-4)}{q(n-2)}\right)}{\left(\frac{q(n-2)}{q(n)}\right)}\right)^{(\frac{1}{2^{2}})}<\left(\frac{\left(\frac{q(n-6)}{q(n-4)}\right)}{\left(\frac{q(n-4)}{q(n-2)}\right)}\right)^{(\frac{1}{2^{4}})}<\cdots
<\left(\frac{\left(\frac{q(n-(s+2))}{q(n-s)}\right)}{\left(\frac{q(n-s)}{q(n-(s-2))}\right)}\right)^{(\frac{1}{2^{s}})}<\cdots<\left(\frac{\left(\frac{q(2))}{q(4)}\right)}{\left(\frac{q(4)}{q(6))}\right)}\right)^{(\frac{1}{2^{(n-4)}})} $
$0\leq s\leq (n-4)$
If $s=(n-4)$ we get the $2^{(n-4)}$'th root of the first monster :
$\left(\frac{\left(\frac{q(2))}{q(4)}\right)}{\left(\frac{q(4)}{q(6))}\right)}\right)^{(\frac{1}{2^{(n-4)}})}$
which by the way don't deserve the name being the nice simple rationel number: $\frac{10}{7}$
But this simplicity will soon come to an end, as n grows.
I was very proud years ago, when I finally proved (with the help of the
harmonic series, I remember) that no matter how big an a
is, sooner or later it will end up being very near 1 if you take the n'th
root of it and make n bigger and bigger.
$a^{\frac{1}{n}}\rightarrow 1, n\rightarrow \infty $
It will be sooner not later if you take the $2^{s}$'th root of $\frac{10}{7}$,
which is not even a big number.
$\left(\frac{10}{7}\right)^{\frac{1}{2^{s}}} \rightarrow 1, s\rightarrow \infty $
If I could prove this suggestion from maple I could forget about my rather
elaborate proof for the monsters jumping or spiraling down to 1
Dark Matter continued: The Case of the Missing Galaxies
August 20th, 2007

Science - 3 Aug 2007, Vol 317, Issue 5838
Science, 3 August 2007, Newsfocus page 594, Where Are the Invisible Galaxies -Adrian Cho.
I am very impressed by the story from 1933:
Some of galaxies in the Coma
cluster moving so fast, that they ought to leave the cluster
And the parallel
story from 1960-70:
The stars in the outskirts of galaxies moving so fast that
they should whirl out in the
emptiness between the galaxies.
There should be a lot of small galaxies of dark matter around but nobody can
find them.
There should be galaxies of ordinary matter marked of the encounter
with galaxies of dark matter but nobody can find them.
I can't help thinking of Boyle's experiment where he (if the story is true) tries to move a feather
with the aether in a box without air.
And Michelson and Morley's trying to show
that an observer moving relative to the aether should obtain different
velocities of the light in the direction of this moving and the perpendicular
one.
The dark matter has the property of invisibility. Maybe it has the property of
not interfering with ordinary matter. Like the Boyle's aether it just moves
through it.
Maybe it has the property of not existing :)
Maybe some day it will be thought unnecessary to explain the problems with the help of the dark matter.
Maybe some day a new Einstein will explain gravity and velocity are intertwined
like the old one explained time and velocity are intertwined as mass and velocity
are.
I just annihilated 90 percent of the universe.
Endangered Species
August 11th, 2007

Hazel Dormouse
Earlier this year there was som focus in my newspaper 'Jyllandsposten' on
a bridge build to cross a highway some place in Jylland or was it Fyn :) to
make the transversal possible for the little Hazel Dormouse (Muscardinus avellanarius from the
family Myoxidae), which is threatened by extinction in Denmark. The project cost severeal millions of DKK.
I was amused and at the same time
touched.
Can't you imagine the nature guard sitting at the end of bridge asking
every passing field vole (Microtus agrestis): 'are you really a hazel dormouse?'
I hope the bridge will be fregvented by a lot of other animals. Since I was young
the only badgers (Meles meles), I have seen are the ones killed by cars at the
side of the road.
Lets hope however they don't meet in the passing, the badger and hazel dormouse, I mean.
Recalling this famous
old touristposter from Copenhagen where a policeman escorts a duck with all her ducklings
over the street, and now the dormouse. Is'nt Denmark a wonderful country, was my thought.

Field Vole
|

European Badger
|

The Delta Smelt
But then I just read about the delta smelt (Hypomesus transpacificus) from
California.
Science, 27 Juli 2007, page 442, Delta Blues, California Style by Robert F. Service.
A California Superior Court have ordered a stop in 10 days of the pumps that send 1/3 of
the water from Sacramento- and San Joaquin rivers back, so it can be used as drinking water and to irrigating in order to give the smelts a pause.It seems the smelts get
trapped in the filters in front of the pumps because the surge from them are
too strong.
If the court makes a habit out of this, then we are not talking about several
million DKK. or even dollars, but billions, if I understand the author of the
article.
Maybe I should correct myself and say: 'what a wonderful world'
Or maybe it's not that amusing or wonderful, maybe its a kind of warning. There may be something wrong in Denmark and the
rest of world. We may not be talking so much of Mice as of Men.
But I am still smiling. So small animals so much commotion.
Sort Stof
August 5th, 2007

Dark Matter
Der er vældig gang i emnet sort stof.
Nogen laver billeder af det, ikke helt
simpelt, når det er usynligt (American Scientist, maj-juni, sd. 258). Blåligt
'frøslim'
lidt spøgelsesagtigt. Spøgelser er jo også næsten usynlige.
Aetherhypotesen genoplivet
August 5th, 2007
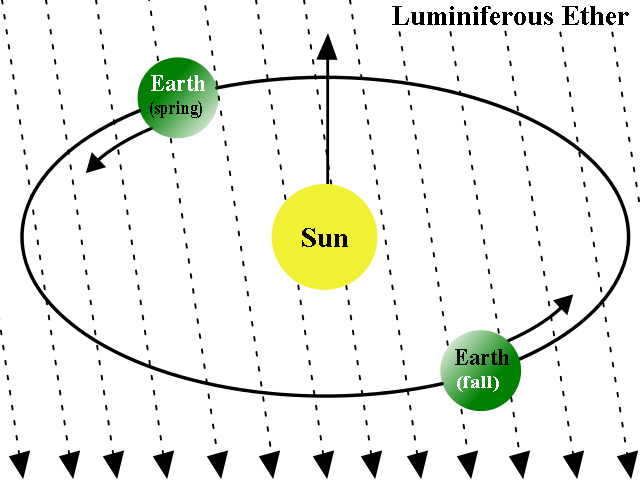
(Science, 6. juli 2007, sd. 32. Racing to Capture Darkness.)
Bedst ville det være om man kunne få stoffet til at interagere med almindelige
velkendte partikler.
Rita Bernabei og hendes team i det italienske DAMA
projekt, mener, at det er lykkedes for dem i et 100 kilogram array af
natriumjodidkrystaller.
Der er endda tegn på årstidsvariationer i antallet af
kollisioner, svarende til, at mælkevejen drejer rundt i en dark matter-suppe,
så jorden f.eks. om sommeren bevæger sig mod vinden og om vinteren med vinden
eller omvendt.
Jeg mener, Boyle gjorde et forsøg på at blæse til en fjer med en lille blæsebælg i
et lufttomt rum, og måtte konstatere at aethervinden var for tynd til påvirke
fjeren. Blæsebælgen kunne måske ikke presse den sammen, den sivede måske gennem
læderet, evt. sivede den gennem fjeren uden at påvirke den.
Ole Rømer opdagede lysets forsinkelse ved hjælp af en måneformørkelse ved
Jupiter registreret på forskellige tidspunkter af året og beregnede lysets
hastighed.
Michelsen og Morleys forsøg på at måle lysets hastighed mod og med
aethervinden burde gentages mod denne nye dark matter-vind.
Problemet er
selvfølgelig, at med og mod i deres forsøg var samtidig, men med lidt fantasi og
hjælp fra en eller anden meget præcis pulsar? Men det er der nok nogen, der har
tænkt på.
Recreated 2024-07-19 by Jakob Schmid